Why Should Students Use A Compass And A Straightedge Instead Of A Drawing Program

Some time ago I looked at questions about trisecting an angle by compass and straightedge, which entailed discussing the rules for such constructions. We left open another common question: Why are such constructions important, and why do we use those particular tools? This probably isn't explained as often as it should be.

Why does it matter? Axioms

I'll start with this question from 1998:

The Importance of Geometry Constructions  I am doing a report on constructions in geometry. I would like to know                why constructions are important. I realize that they challenge us to use different tools but there must be more to it then that. So I was wondering if you could give me more of a reason why constructions are so important?

Since many things we ask children to do are largely to get them used to certain ways to use their hands or bodies, it is understandable that Kel would suppose that we teach constructions just because compasses and straightedges are worth knowing how to use. But that isn't really it. Ultimately, it's because our minds are worth knowing how to use!

I answered:

Hi, Kel. That's a good question. We tend to teach it out of tradition, and forget to think about why it's worth doing!  Certainly learning how to use the tools is useful. Some of the techniques are useful in construction (of buildings, furniture, and so on), though in fact sometimes there are simpler techniques builders use that we forget to teach. But I think                the main reason for learning constructions is their close connection to axiomatic logic. If you haven't heard that term, I'm talking about the whole idea of proofs and careful thinking that we often use geometry to teach.

I've used compass constructions when I helped renovate a church building; but then the "compass" was a length of string. It was the idea behind it that really mattered.

Euclid, the Greek mathematician who wrote the geometry text used for centuries, stated many of his theorems in terms of constructions.                His axioms are closely related to the tools he used for construction.                Just as axioms and postulates let us prove everything with a minimum of assumptions, a compass and straightedge let us construct everything precisely with a minimum of tools. There are no approximations, no guesses. So the skills you need to figure out how to construct, say, a square without a protractor, are closely related to the thinking skills you need to prove theorems about squares.

A construction is, at root, a theorem: If you follow this sequence of steps, the result will necessarily be the object you claim to be creating, such as the bisector of an angle, or a triangle that meets certain requirements. So learning to design a construction is practice in "constructing" geometrical proofs. Practice in construction is not primarily practice in using your hands, but your mind.

I closed my short answer by referring to the first proof in Euclid's Elements, Proposition I.1:

Proposition 1

To construct an equilateral triangle on a given finite straight line.

LetAB be the given finite straight line.

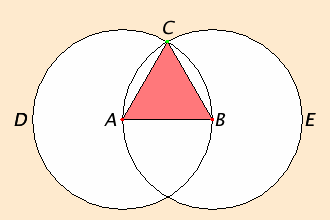
It is required to construct an equilateral triangle on the straight lineAB.

Describe the circleBCD with centerA and radiusAB. Again describe the circleACE with centerB and radiusBA.Join the straight linesCA andCB from the pointC at which the circles cut one another to the pointsA andB.

Now, since the pointA is the center of the circleCDB, thereforeAC equalsAB. Again, since the pointB is the center of the circleCAE, thereforeBC equalsBA.

ButAC was proved equal toAB, therefore each of the straight linesAC andBC equalsAB.

And things which equal the same thing also equal one another, thereforeAC also equalsBC.

Therefore the three straight linesAC, AB, andBC equal one another.

Therefore the triangleABC is equilateral, and it has been constructed on the given finite straight lineAB.

Q.E.F.

This theorem is in reality a construction. Note that the steps involve making circles (with a compass) and making lines (with a straightedge); and at the end he puts "Q.E.F.", short for "Quod erat faciendum", Latin for "Which was to be done". (Euclid, of course, actually used Greek, "ὅπερ ἔδει ποιῆσαι", "hoper edei poiēsai".)

Why not rulers and protractors? Axioms

In 2002, we got a similar question from a teacher, that called for a little more detail on how the axioms (Euclid's Postulates) relate to the compass and straightedge:

Why Straightedge and Compass Only?  My Geometry students want to know                why constructions can only be done using a straightedge and a compass. They want to know why they can't just measure a line segment to copy it or use a protractor to construct an angle. What's the difference?  We have searched our book as well as some internet sites containing constructions, but to no avail.

I referred back to the previous answer, then elaborated.

There are two ways that I can see to explain the restrictive rules for constructions, which come to us from the ancient Greeks:  1. They are just                the rules of a game mathematicians play. There are many other ways to do constructions, but the compass and straightedge were chosen as one set of tools that                make a construction challenging, by limiting what you are allowed to do, just as sports restrict what you can do (e.g. touching but not tackling, or tackling but no nuclear weapons) in order to keep a game interesting. Other tools could have been chosen instead; for example, geometric constructions can be done using origami.

Euclid could have started with any tools he wanted; but a major goal was to restrict what could be done, as sort of a game to see how little we can use, to do how much.

For more on axioms or postulates, see my series in July 2018, beginning with Why Does Geometry Start With Unproved Assumptions?

But it's not just a game; it's the game:

2. They are the basis of an axiomatic system, with the goal of ensuring that geometry is built on a solid foundation. Euclid wanted to start with as few assumptions as possible, so that all of his conclusions would be certain if you just accepted those few things. So he listed five postulates (in addition to some other assumptions even more basic); I've taken these from the reference given in my answer above:    Postulate 1.    [It is possible]                to draw a straight line                from any   point to any point.     Postulate 2.    [It is possible]                to produce a finite straight line                                continuously in a straight line.    Postulate 3.    [It is possible]                to describe a circle                with any   center and radius.     Postulate 4.    That all right angles equal one another.     Postulate 5.    That, if a straight line falling on two straight   lines makes the interior angles on the same side   less than two right angles, the two straight lines,   if produced indefinitely, meet on that side on   which are the angles less than the two right angles.

He starts with the existence of lines and circles, then adds only two additional facts. (His system is not quite complete, and additional axioms are now known to be necessary.)

The first two postulates say that you can use a                straightedge: line it up with two given points, and draw the line between them, or line it up with an existing segment, and draw the line beyond it. That's the first tool you are allowed to use, and those are the only ways you are allowed to use it.

As is often noted, you are not allowed to do other things, like measure or copy a length by making marks on the straightedge. This is not just because Euclid wanted to keep his tools clean! It's because he wanted to minimize his assumptions, proving as much as possible starting with as little as possible.

The third postulate says you can use a                compass                to draw a circle, given the center and radius (or a point on the circle). That is the only way you are allowed to use the compass; you can't, for example, draw a circle tangent to a line by adjusting its radius until it _looks_ tangent, without knowing a specific point the circle has to pass through.  The last two postulates relate to angles, and are less associated with the construction process itself than with what you see when you are done.

Again, the restrictions are to minimize the assumptions, not because his compass was defective. I actually understated the restriction in this case. (More on that later.)

So really the two tools Euclid required for a construction just represent the assumptions he was willing to make: if these two tools work, then you can construct everything he talks about. For example, you can use these tools, in the prescribed manner, to construct a tangent to a given circle through a given point; but it takes some thought to find how to do so (without just drawing a line that _looks_ tangent), and it takes several theorems to show that it really works.

Here we are back to the challenge! And the goal is not just to make something that looks right, but to be able to prove something.

Of course you CAN just measure a line or an angle, if your goal is just to make a drawing - and                usually that will be more accurate than a complicated compass construction! But when you use only the tools allowed in this game, you are actually playing within an axiomatic system, getting a feel for how proofs work. You are simultaneously playing a challenging game, and doing one of the few things in life that can give you absolute certainty:                if these lines and circles were exactly what they pretend to be (with no thickness, etc.), then the point I construct would be exactly what I claim it is. And it's that sense of certainty that the Greeks were looking for.

Why does the compass collapse? Axioms!

I didn't mention above a special restriction on the compass, which turns out to be entirely theoretical. We got a question about that in 2003:

Collapsible Compass  I need to know                what a collapsible compass is                and what it is used for. All I know is that when you pick it up from the paper, you lose your place.

Again, I answered the question, keeping it brief:

The collapsible compass is not something that is "used"; rather, it represents the fact that Euclid wanted to make as few assumptions (postulates, or axioms) at the base of his proofs as possible. So rather than assume that it was possible to move a line around, keeping the same length (as you could do with a real, fixed compass), or equivalently that you can draw a circle with a given center and length,                he assumed only that you can draw a circle with a given center and through a given point. Then he went on to prove that if you could do that, you COULD then construct a circle with a given radius, or move a line to a given place:    Collapsible Compass   http://mathforum.org/library/drmath/view/52601.html

The reference is to a short answer that links to the proposition I am about to discuss.

Where I quoted Euclid's postulates above, it may look as if you can just set the compass to any radius you want, contrary to what I've said here: "[It is possible] to describe a circle with any center and radius." But the word "radius" to Euclid does not refer to a number, as we think of it today, but to a specific segment! This is made explicit in the commentary to the Elements on Joyce's site that I've referred to before, Postulate 3:

Circles were defined in Def.I.15 and Def.I.16 as plane figures with the property that there is a certain point, called the center of the circle, such that all straight lines from the center to the boundary are equal. That is, all the radii are equal.

The given data are (1) a point A to be the center of the circle, (2) another point B to be on the circumference of the circle, and (3) a plane in which the two points lie. …

Note that this postulate does not allow for the compass to be moved. The usual way that a compass is used is that is is opened to a given width, then the pivot is placed on the drawing surface, then a circle is drawn as the compass is rotated around the pivot. But this postulate does not allow for transferring distances. It is as if the compass collapses as soon as it's removed from the plane. Proposition I.3, however, gives a construction for transferring distances. Therefore, the same constructions that can be made with a regular compass can also be made with Euclid's collapsing compass.

Here is Proposition I.3:

Proposition 3

To cut off from the greater of two given unequal straight lines a straight line equal to the less.

In the construction, Euclid effectively draws a circle whose radius is the length of a given segment located elsewhere. In order to do this, he uses Proposition I.2:

Proposition 2

To place a straight line equal to a given straight line with one end at a given point.

This is equivalent to setting a compass to the length of the given segment. So with these propositions, in effect we have used the "collapsible compass" to make a new, better compass that doesn't collapse.

Continuing with my answer,

Once that was proved, you didn't have to use a collapsible compass, but could use a regular one, knowing that                any construction you could do this way, you could do with a collapsible compass.  Note that a real "collapsible compass" would not work, because the radius would change as you drew the supposed circle! The idea is that it holds together and keeps its radius as long as the center point is in place, but loses it when you pick it up. I don't know of any design for a real-world compass that would work that way;                this is a tool that exists only in the mind of a geometer, as a description of Euclid's postulate 3.

Can real compasses collapse?

Let's close with a question from 2011, asking about the history of real compasses:

The Collapse of Compasses that Do Not Copy Segments, and the Lengths We Go To http://mathforum.org/library/drmath/view/76724.html  Having studied both ancient Euclidean geometry and more recent treatments of the subject, I am wondering exactly                when it was that compasses began to be used to copy lengths.  I have read that Euclid disallowed compasses from doing this because in his geometry there was no motion. To me, this restriction makes a lot of sense both from a practical and a theoretical viewpoint: one cannot set the compass at a certain length and then truly claim the ability to duplicate that length anywhere other than at a segment radiating from its current center. Anyway, Euclid covers this problem in his second proposition.  But in our current geometry textbook, my students and I encounter "Copying a segment is easy:                we just set the compass to the length of the segment, and then copy it elsewhere on the page." Perhaps this concession comes only after centuries' worth of students saying, "Why can't we just measure the length and then copy it?" Obviously, this does make a certain type of sense, but to me it seems a sour departure from Euclid's impregnable logic.  What really confuses me is that Euclid already HAD a solution to this problem. Granted, that construction is somewhat involved, even difficult -- certainly out of proportion for such a simple objective. But not only does it WORK, it maintains our ability to do consistent geometry without introducing this vague notion of "moving" lengths.  My current work on this problem involves the simple observation that when we move compasses, they often shift slightly. Unless we have a drafting compass or something, the "pick-up-the-compass-and-move-it" part will usually involve some alteration in the setting of the compass, however imperceptible.

As I've said, it was never really about physical compasses. I referred to the page above and continued:

I can't say that I know anything about the history of compasses. Many modern compasses (and likely many old ones) can hold their settings reasonably well. Since                using them that way is simply a shortcut to something that CAN be done with the collapsible compass                assumed in Euclid's postulates (a la Proposition 2, to which you refer), to require students not to trust them to do this would make constructions a terrible chore, and                would not make math any more understandable, much less enjoyable.

We don't have to restrict ourselves, because the nature of the compass is nothing more than a reflection of an axiom. (The ones we use in class reflect a theorem instead!)

So how should we teach students about this?

In a course built around postulates, I would certainly mention that the postulate does not in itself allow transferring a length using a compass, but that we can use that process as a                shortcut to a longer procedure                that could be done with the so-called collapsible compass. But                I would not subject students to more than one exercise in which they actually had to perform the long procedure of copying a segment!                Once they know it can be done, doing it would be a waste of time. I imagine that may have been true even in Euclid's time.

Mathematicians make jokes about their propensity to skip over anything that has been "reduced to a previously solved problem". Once you know you could do it with a collapsing compass, you are allowed to do it directly.

But there's some reality there, too:

Of course I should note that there are also many (cheaper)                compasses that don't hold their settings well enough to make even a single circle accurately! In my math course for elementary teachers, most of them buy a compass of poor quality, and I have to take some time to teach them how to use it so that it will retain its setting (which involves holding the compass in such a way that all forces are perpendicular to the direction in which it opens). This is probably a good thing for teachers to have to learn! For high school students, good compasses would be a good investment.

But those cheap compasses don't just collapse between circles like Euclid's imaginary compass; they slip even as you draw! Never use them for anything that matters.